 microseminar
microseminar
informal seminar of Doppler Institute
on quantum theory and on the related topics and methods.
(1) not too frequently,
just from time to time,
this meeting takes place
on the second floor of the
Nuclear Physics Institute
in Rez
in the seminar room of the
Department of Theoretical Physics.
Presented strictly in a micro style, i.e.,
lasting 20 minutes and followed by unlimited discussion.
(2) more frequently,
in a way announced
on
a separate webpage
and in the more standard style designed and controlled
by Jiri Lipovsky,
the upgraded seminar is more regular, taking place
in the University of Hradec Kralove
usually on Thursdays, around 10.00 a.m.,
usually
in the
briefing room of the Faculty of Science
on the fourth floor
of the S-building,
usually accessible also in hybrid form using
Zoom.
Note added:
interested speakers
are always warmly
welcome.
FORTHCOMING SESSION IN REZ:
| Place: |
t. b. a.
|
| Time: | t.b.a. (just the duration is fixed: 20 minutes + questions) |
| Date: |
|
| Speaker: |
|
| Title: |
|
Abstract: | t. b. a. |
Remark: |
Zoom connection, if available, will be announced in due time |
FORTHCOMING SESSION IN HRADEC,
with the more detailed announcement available
on the new dedicated webpage
:
| Place: | Hradec Kralove,
UHK, briefing room Nr 74060 of the Faculty of Science (the fourth floor of the S-building)
|
| Time: | 10:00 a.m. (duration: 20 minutes + questions) |
| Date: |
|
| Speaker: |
|
| Title: |
|
Abstract: | Motivated by the EPR paradox version in the measurement of a quantum particle position, a simplified one-dimensional model of measurement of the particle position by two distant detectors is considered. Detectors are modelled by quantum particles bounded in potential wells with just two bound states, prepared in the excited states. Their de-excitation due to the short range interaction with the measured particle is the signal for the detection. In the approximations of short time or small coupling between particle and the measuring apparatuses, the simultaneous detection of the particle by both detectors is suppressed. The talk is based on J. Dittrich, Acta Polytechnica 62 (2022), 445. |
Remark: |
Zoom connection |
ARCHIVE:
| Place: |
UHK, briefing room Nr 74060 of the Faculty of Science (the fourth floor of the S-building)
|
| Date and time: |
Thursday, March 2nd, 2023,
10:45 a.m. -- 11:15 a.m.
|
| Speaker: | Vladimir Lotoreichik (NPI ASCR, Rez) |
| Title and abstract: |
Bound states of weakly deformed soft waveguides
|
Spectral analysis of the two-dimensional Schrodinger operator with an attractive potential being a multiple of the characteristic function of an
unbounded strip-shaped domain was presented. The Schrodinger operator with such a potential
describes the recently proposed model of a soft quantum waveguide. The thickness of the strip-shaped domain is varying and given by the function d + ef(x),
where d is a positive constant, f is a continuous, compactly supported function
on the real line, and e is a positive small parameter. Our main results concern
the negative discrete spectrum of this Schrodinger operator in the limit e -- > 0. In
particular, we generalize the weak deformation asymptotics obtained for Dirichlet
tubes by Bulla, Gesztesy, Renger, and Simon to the setting of soft quantum waveguides.
The results were obtained in collaboration with Pavel Exner and Sylwia Kondej. |
| Remark: |
The talk was not recorded. Besides
participants onsite,
connection by Zoom was also available.
|
| Place: |
UHK, briefing room Nr 74060 of the Faculty of Science (the fourth floor of the S-building)
|
| Date and time: |
Tuesday, January 31st, 2023,
10:45 a.m. -- 11:30 a.m.
|
| Speaker: | Florian Oschmann (Institute of Mathematics CAS, Prague) |
| Title and abstract: |
Stratified fluids: On pancakes and non-local temperatures
|
We investigate the rigorous derivation of two models for incompressible fluid flow: a) Majda's
''stack of pancakes'' model, governing essentially two-dimensional flow with momentum coupling
in the vertical variable; b) the Oberbeck-Boussinesq approximation, governing the motion
of a heat conducting fluid heated from below and cooled from above. Their derivation from
the full compressible Navier-Stokes-Fourier system has been done for a) the inviscid (Euler)
case where no coupling occurs, and b) the case of Neumann BC for the temperature. We will
investigate the rigorous justification of both models for the case of viscous fluids with temperature
Dirichlet BC, and show that a) indeed there is vertical coupling, and b) an unexpected
temperature term arises.
Joint work with Peter Bella (TU Dortmund), Eduard Feireisl (CAS), Danica Basari\'c (CAS), and Edriss S. Titi (Uni Cambridge). |
| Remark: |
besides
participants onsite,
connection by Zoom was
also used
|
| Place: |
Prague, Narodni trida 3, building of AV CR,
lecture hall Nr. 205 (exceptional)
|
| Date and time: |
Thursday, January 5th, 2023,
11:00 a.m. -- 12:00 a.m.
|
| Speaker: | David Krejcirik (FNSPE CTU, Prague) |
| Title and abstract: |
Is the optimal rectangle a square?
| We give a light talk on an optimality of a square in geometry and physics. First, we recollect classical geometric results that the square has the largest area (respectively, the smallest perimeter) among all rectangles of a given perimeter (respectively, area). Second, we recall that the square drum has the lowest fundamental tone among all rectangular drums of a given area or perimeter and reinterpret the result in a quantum-mechanical language of nanostructures. As the main body of the talk, we present our recent attempts to prove the same property in relativistic quantum mechanics, where the mathematical model is a matrix-differential (Dirac) operator with complex (infinite-mass) boundary conditions. It is frustrating that such an illusively simple and expected result remains unproved and out of the reach of current mathematical tools. |
| Remark: | connection by Zoom was not
available; ask
participants onsite;
|
| Place: | exceptionally in the room S3 (the ground floor of the UHK S-building) |
| Date and time: |
Thursday, November 10th, 2022,
10:45 -- 11:15 a.m.
|
| Speaker: | Bekir Can Lutfuoglu (UHK) |
| Title and abstract: |
Dunkl oscillators
| The concept of symmetry has an undeniable role in physics. Dunkl operators, which are expressed by the combination of differential and difference operators, enable simultaneous examination of parity(reflection symmetry) in solutions of physical systems. In this talk, we will handle relativistic and nonrelativistic Dunkl oscillators and briefly discuss parity-dependent solutions. |
| Remark: | hybrid form, connection by Zoom was available as usual |
| Place: | briefing room of the Faculty of Science (4th floor), UHK |
| Date and time: |
Thursday, October 6th, 2022,
11:45 a.m. -- 12:15 p.m.
|
| Speaker: | Mauricio Pato (Universidade de Sao Paulo, Brasil) |
| Title and abstract: |
Dyson map and the
pseudo-Hermitian entanglement of
random states
| In a recent paper (A. Fring and T. Frith, Phys. Rev A 100, 101102 (2019)), Dyson’s scheme to deal with density matrix of non- Hermitian Hamiltonians has been used to investigate the entanglement of states of a PT-symmetric bosonic system. They found that von Neumann entropy can show a different behavior in the broken and unbroken regime. We show that their results can be recast in terms of an abstract model of pseudoHermitian random matrices. It is found however that, although the formalism is practically the same, the entanglement is not of Fock states but of Bell states. |
| Remarks: | participants onsite; video-recorded version of the talk is available upon emailed request |
| Place: | lecture hall S2 of the Department of Physics (UHK) in Hradec Kralove |
| Form: | Zoom connection was not available this time |
| Date and time: |
Monday, September 12th, 2022,
11:45 a.m. -- 12:45 p.m.
|
| Speaker: | Ali Mostafazadeh (Koc University, Istanbul) |
| Title and abstract: |
Renormalization of point scatterers in two and three
dimensions and its coincident-limit problem
| In two and three dimensions, the standard treatment of the scattering problem for a multi-delta-function potential, ??(??)=????? ??(??-????),??1 leads to divergent terms. Regularization of these terms and renormalization of the coupling constants ???? give rise to a finite expression for the scattering amplitude, but this expression has an important short-coming; in the limit where the centers ???? of the delta functions coincide, it does not reproduce the formula for the scattering amplitude of a single-delta-function potential, i.e., it seems to have a wrong coincidence limit. We provide a critical assessment of the standard treatment of these potentials, offer a resolution of its coincidence-limit problem, and reveal its inability to determine the dependence of the scattering amplitude on the distances between the centers of the delta functions. This is in sharp contrast to the treatment of this problem offered by a recently proposed dynamical formulation of stationary scattering (DFSS). For cases where the centers of the delta functions lie on a straight line, this formulation avoids singularities of the standard approach and yields an expression for the scattering amplitude which has the correct coincidence limit. |
| Remark: |
participants onsite
|
| Place: | lecture hall S2 of the Department of Physics (UHK) in Hradec Kralove |
| Form: | hybrid form: onsite plus Zoom |
| Date and time: |
Thursday, May 19th, 2022,
10:45 a.m.
|
| Speaker: | Iveta Semoradova (NPI Rez) |
| Title and abstract: |
Diverging eigenvalues in domain truncations of Schroedinger operators with complex potentials.
|
Domain truncations of Schroedinger operators with complex potentials are known to be spectrally exact. However, several examples suggest that additional eigenvalues escaping to infinity seem to be a generic feature. We find conditions on the presence of such eigenvalues and obtain their asymptotic expansions. Our approach also yields asymptotic formulas for diverging eigenvalues in a strong coupling regime for the imaginary part of the potential.
The talk was based on joint work with P. Siegl (Graz). |
| Remark: |
participants
|
| Place: | communicated, by Zoom, from the Department of Physics (UHK) in Hradec Kralove |
| Form: | online only |
| Date and time: |
Thursday, April 28th, 2022,
10:45 a.m.
|
| Speaker: | Hassan Hassanabadi (UHK) |
| Title and abstract: |
Investigation of the Dunkl oscillator in the position and momentum
representation
| In this work, first we introduce Dunkl operator and its properties as well as $\nu$-deformed exponential function. Then the Dunkl-Heisenberg relation in the position and momentum representation is obtained by applying the reflection operator for the position and momentum. We then obtain the corresponding eigenfunction and eigenvalue. Based on the $\nu$-deformed Hermite polynomials, we derive the energy levels, ground-state wave functions, and excited wave functions. We expand the method to obtain the related coherent states. |
| Remark: |
participants
|
| Place: | lecture hall S2 of the Department of Physics (UHK) in Hradec Kralove |
| Form: | hybrid form: onsite plus Zoom |
| Date and time: |
Thursday, March 24th, 2022,
10:45 a.m.
|
| Speaker: | Petr Siegl (TU Graz) |
| Title and abstract: |
The damped wave equation with singular damping
|
We analyze the spectral properties and peculiar behavior of solutions of a damped wave equation on a finite interval with a singular damping of the form \alpha/x with a positive \alpha. We establish the exponential stability of the semigroup and determine conditions for the spectrum to consist of a finite number of eigenvalues. As a consequence, we fully characterize the set of initial conditions for which there is extinction of solutions in finite time.
The talk was based on joint work with P. Freitas (Lisbon) and N. Hefti (Bern). |
| Remark: |
participants
|
| Place: | lecture hall S2 of the Department of Physics (UHK) in Hradec Kralove |
| Form: | hybrid form: onsite plus Zoom |
| Date and time: |
Thursday, March 3rd, 2022,
10:45 a.m.
|
| Speaker: | Anton Galaev (UHK) |
| Title and abstract: |
Holonomy groups and their application
| A short introduction to the holonomy groups of linear connections on smooth manifolds will be given. I will explain the classification results for the Levi-Civita connection on Riemannian and Lorentzian manifolds as well as for the metric connections with skew-symmetric torsion. The importance of these results is shown by the fact that spaces with a broad class of holonomy groups automatically satisfy the Einstein equation and admit parallel spinor fields (supersymmetries). |
| Remark: |
participants
|
| Place: | University of Hradec Kralove, Faculty of Science |
| Form: | online talk, presented by Zoom |
| Date and time: |
Thursday, February 3rd, 2022,
10:45 a.m.
|
| Speaker: | Jan Kriz (UHK) |
| Title and abstract: |
Soft quantum waveguides
| A brief review of the theory of planar quantum waveguides will be provided. The recent results concerning so-called soft waveguides will be discussed in more details. Mathematically, we investigate the existence of discrete spectrum of two-dimensional Schrodinger operators with an attractive potential in the form of a channel of a fixed profile built along an unbounded curve. |
| Remark: |
participants
|
| Place: | University of Hradec Kralove, Faculty of Science, Department of Physics, Building S, lecture hall S2 |
| Form: | talk presented in the hybrid (i.e., Zoom + onsite) form this time |
| Date and time: |
Thursday, January 6th, 2022, 10:45 a.m.
|
| Speaker: | Jiri Lipovsky (UHK) |
| Title and abstract: |
Spectral determinants
|
We introduce a generalization of the notion of the determinant from matrices to operators with infinitely many eigenvalues, which uses the spectral zeta function. We show how to compute it for simple operators and sketch the main difficulties in its definition, especially the dependence of the spectral determinant on the branch cut of the logarithm appearing in the definition of the spectral zeta function.
This is joint work with P. Freitas based on the paper [1]. [1] P. Freitas, J. Lipovsky, Spectral determinant for the damped wave equation on an interval, Acta Physica Polonica A 136 (2019), 817-823 [arXiv: 1908.06862 [math-ph]]. |
| Form: | virtual this time: Jan Kriz (UHK) invited us to join via Zoom |
| Date and time: |
Thursday, December 2nd, 2021, 10:45 a.m.
|
| Speaker: | Marzieh Baradaran (UHK) |
| Title and abstract: |
Spectrum of a Kagome network
|
Spectral properties of quantum graphs in the form of a kagome or a triangular lattice
were investigated
assuming that wave functions at the vertices are matched through conditions which are non-invariant with respect to the time-reversal. While the positive spectrum consists of an infinite number of bands, the negative one has at most three and two bands, respectively. We discussed, in particular, the high-energy behavior of such systems and the probability that a randomly chosen positive number belongs to the spectrum.
This is joint work with Prof. Pavel Exner based on preprint [1]. [1] M. Baradaran, and P. Exner: arXiv:2106.16019 [math-ph] |
| Place: | University of Hradec Kralove, Faculty of Science, Department of Physics, Building S, lecture hall S3 |
| Date and time: |
Thursday, November 4th, 2021, 11:00 a.m.
(triple length: 60 min.) |
| Speaker: | Petr Seba (UHK) |
| Title and abstract: |
Applications of repetitive ballistographic data
| Mathematically processed ballistography signals are used to evaluate various clinically significant markers routinely used in the human diagnostics. |
| Place: | University of Hradec Kralove, Faculty of Science, Department of Physics, Building S, laboratory S19 on the second floor |
| Date and time: | Tuesday, October 12th, 2021, 10:45 a.m. |
| Speaker: |
Andrii Khrabustovskii (UHK) |
| Title and abstract: | Construction of self-adjoint differential operators with prescribed spectral properties |
The objective of the talk is to illustrate how one can construct domains or potentials such that the essential or discrete spectrum of Laplace- and Schroedinger-type operators coincides with a predefined subset of the real line. Another aim is to emphasize that the spectrum of a differential operator on a bounded domain or bounded interval is not necessarily discrete, e.g., eigenvalues of infinite multiplicity or continuous spectrum may be present.
The talk is based on resent works with J. Behrndt (TU Graz). |
| Place (unusual!): | University of Hradec Kralove, Faculty of Science, Department of Physics, Building S, ground floor, room Nr. S13 |
| Date and time: | Thursday, September 16th, 2021, 10:30 a.m. |
| Speaker: |
Miloslav Znojil (UHK) |
| Title and abstract: | Alice in the wanderland of algebraic geometry, and behind quantum catastrophes | A compact reparametrization of the Arnold polynomial potentials of arbitrary degree (known from the classical theory of catastrophes) is introduced and shown useful in quantum phenomenology. Main attention is paid to the specific unstable-localization dynamical regime. In this regime a systematic determination of the observable density distributions proves feasible via 1/N approximation yielding a qualitative classification and approximate description of a new type of quantum phase transitions, tractable as the (avoided) level crossings or, alternatively, as a manifestation of the existence of a fairly close complex exceptional point. |
| Place: | University of Hradec Kralove, Faculty of Science, Department of Physics, Building S, 1st floor, room Nr. 72549 |
| Date and time: | Thursday, June 17th, 2021, 10:30 a.m. |
| Speaker: |
Miloslav Znojil (UHK) |
| Title and abstract: | From Cizek and Dyson to Bender | In the first microseminar organized in UHK the Bender-inspired formulation of quantum mechanics (a.k.a. PT-symmetric quantum mechanics) will be briefly reviewed. With emphasis on the history of the field, five related paradoxes will be formulated. (1) People never noticed the overlap of the theory with the Jiri Cizek's coupled cluster method in which one calculates the real spectrum using realistic Hamiltonians in non-Hermitian representation. (2) Only too late people noticed the overlap of the theory with an analogous Freeman Dyson's recipe. (3) Frequent misunderstandings were evoked by the Bender's claim that his Hamiltonians were non-Hermitian. At present, it is clear that they are only non-Hermitian in an unphysical, auxiliary, irrelevant Hilbert space. In this sense, their proper name should have been quasi-Hermitian Hamiltonians. (4) The main inspiration of the Bender's new theory was the Bessis' imaginary cubic interaction model. In 2012, in a paper by Siegl and Krejcirik, this particular model was proved not to fit the theory. (5) Subsequently, the initial orientation of the theory on the so called closed quantum systems has changed. Today, most people apply the concept of PT symmetry to open quantum systems. |
| Photo of whiteboard: |
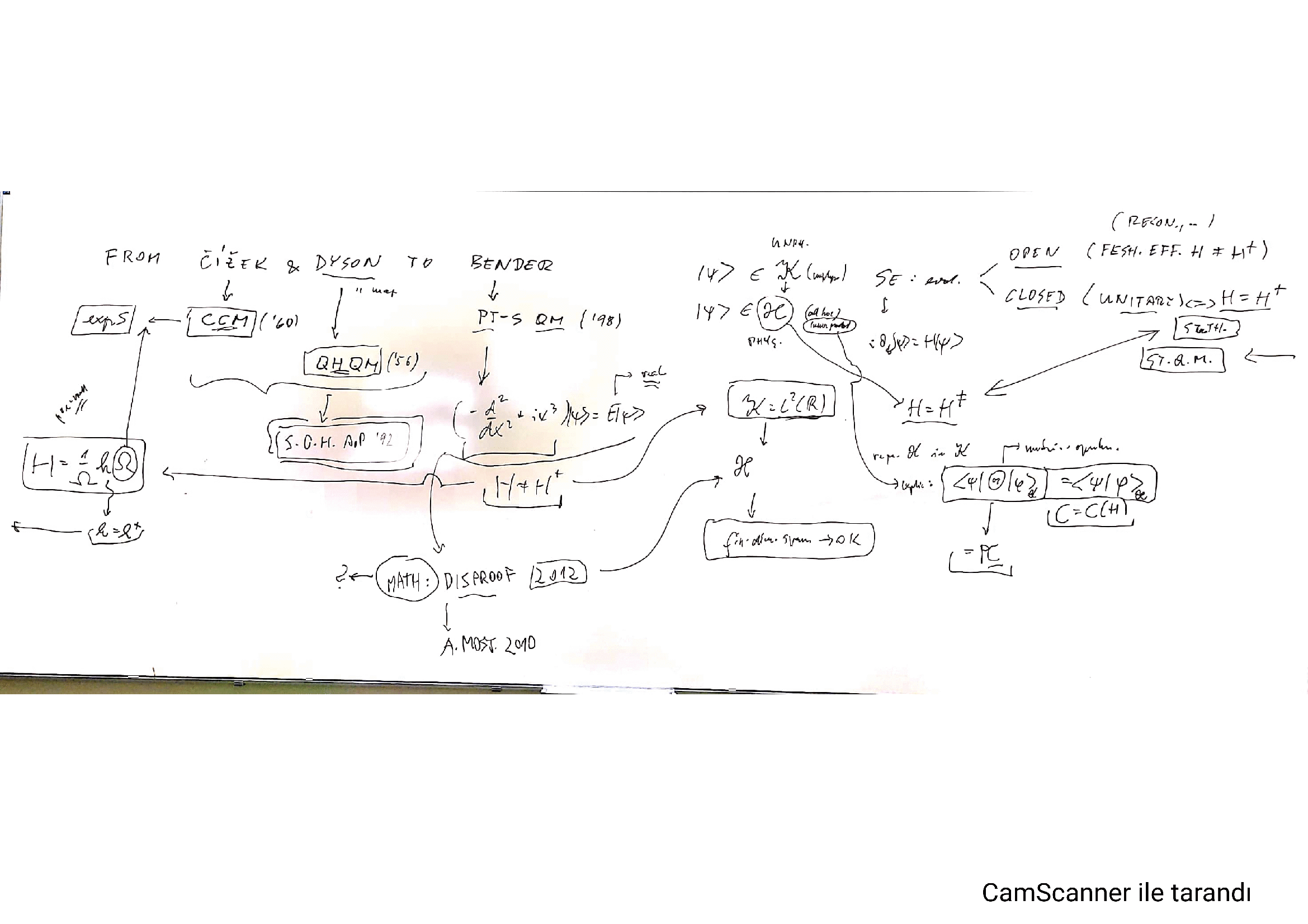
|
The archive of the older microseminars:
The list of talks during 2016 - 2020
The list of talks during 2012 - 2015
The list of talks during 2008 - 2011
The list of the talks during 2008
A compactified list of the speakers during 2008
The list of the talks during 2007
A compactified list of the speakers during 2007
The list of the talks during 2006
A compactified list of the speakers during 2006
PS: in parallel, nested seminars of the similar type may be also sought on the webpages of our local
microconferences
devoted to the analytic an algebraic methods in physics
Info for interested speakers:
|
send email to
Miloslav Znojil | |
| propose a talk (Thursdays on 10.30 or 10:45 are preferable) |
|
on any subject related to
|
|
| you should book your term of talk: | not later than 2 or 3 days in advance |
| your talk's length should be | 20 minutes |
| time for subsequent questions: | unlimited |
return upwards